Mathematicians solve long-standing geometry problem on equiangular lines
For more than 50 years, mathematicians have been pondering a problem in discrete geometry concerning equiangular lines — how many such lines can exist simultaneously in a given dimension?
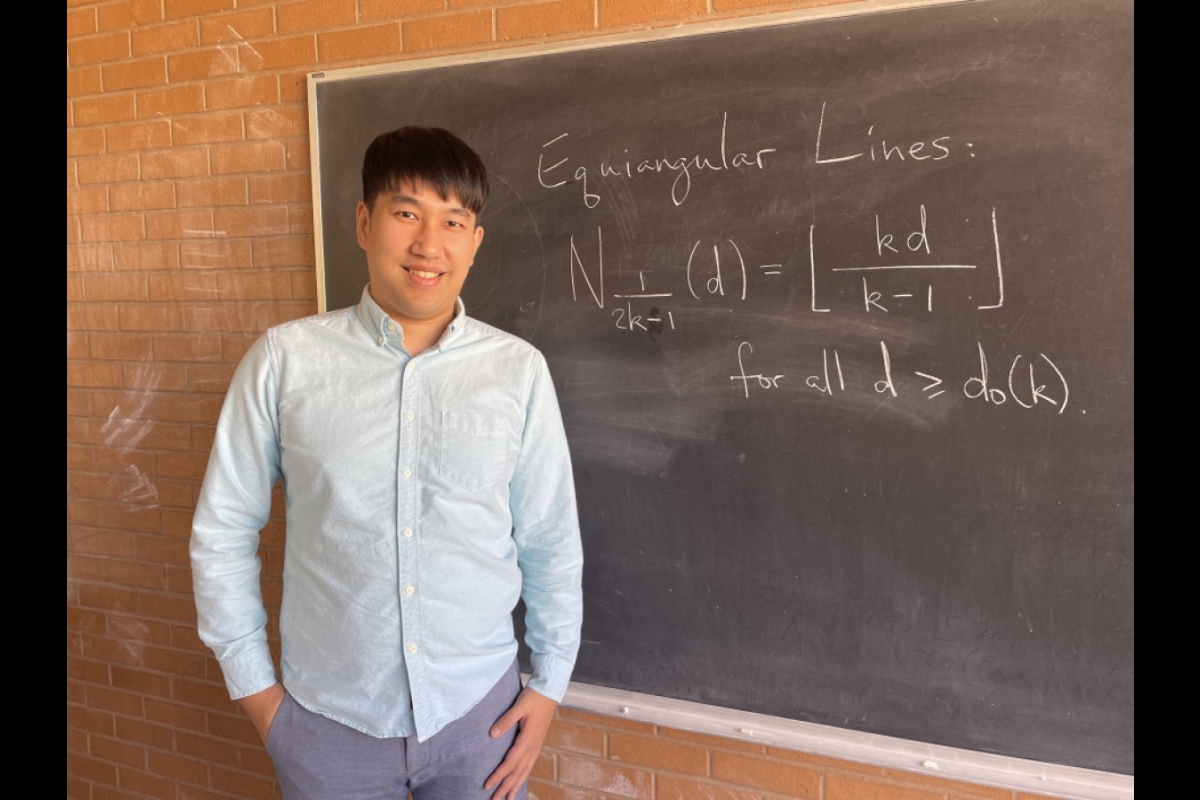
Zilin Jiang joined Arizona State University in January 2021 with a joint position as assistant professor in the School of Mathematical and Statistical Sciences and the School of Computing and Augmented Intelligence. Jiang and a team of researchers at MIT recently solved this long-standing problem with the results published in the Annals of Mathematics, “Equiangular lines with a fixed angle.”
Illustration by Zilin Jiang
Download Full Image
What are equiangular lines?
Jiang explains: “Try to imagine all kinds of ways of arranging lines in space, but the particular arrangements we are interested in are the arrangement of lines so that they are pairwise separated by the same angle. Here are two examples. In a two-dimensional plane, you can think about a regular hexagon and three diagonal lines that pairwise are separated by 60 degrees. Then you can ask the same question in three dimensions — you want to place as many lines as possible in three-dimensional space so that the lines are pairwise separated by the same angle. It turns out the best way to do that is with a regular icosahedron, which has 12 vertices, so that you can pair them together to form six diagonal lines which are separated by the same angle.”
“As mathematicians, we ask the question for two dimensions, then three dimensions, and then we start asking about high dimensions. And that’s the problem we wanted to solve,” said Jiang.
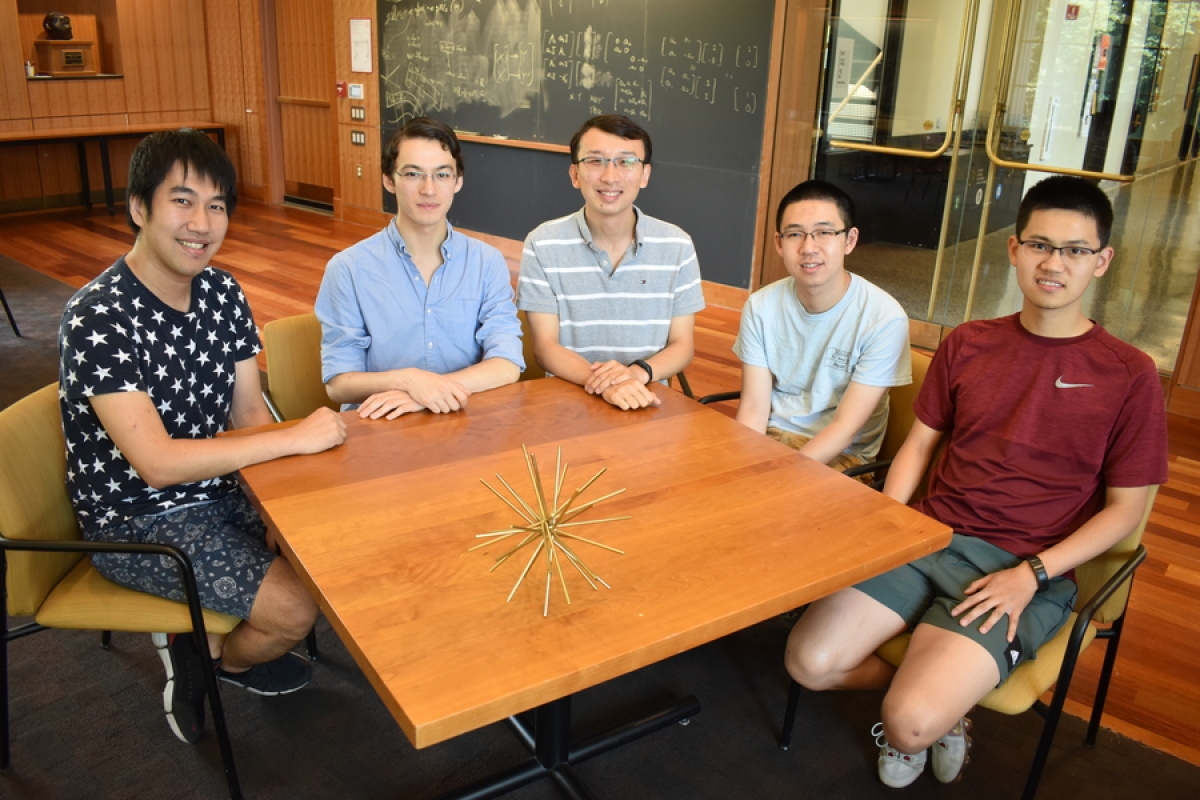
Before coming to ASU, Jiang was a postdoctoral researcher at MIT, working with Assistant Professor Yufei Zhao. Along with Zhao’s PhD student and two undergraduate students, the group of five teamed up to work on the problem as a summer research project.
The problem dates back to the 1970s and has a connection to an area of math called elliptic geometry. There were some early developments on the problem of equiangular lines, but then it became dormant for a while. When Jiang was a graduate student at Carnegie Mellon, his PhD adviser, Boris Bukh, took a look at the problem again.
“He likes to look into the literature and see if the modern techniques can shed new light on the problem, and he made some progress in that direction,” said Jiang.
Jiang read Bukh’s paper, noting there are still cases that are wide open in higher dimensions. He tried to make some further progress on it, but as a graduate student, he didn’t manage to do anything — but the problem was sitting in the back of his mind.
“Some people say there are little people in your brain that just work on the problem secretly and quietly, without you noticing it,” said Jiang, as he gestures with his fingers moving back and forth like little legs dangling behind his head. “Fast-forward maybe one year later, I was a postdoc at Technion – Israel Institute of Technology, and I saw this new work by Benjamin Sudakov, Peter Keevash, Igor Balla and Felix Dräxler, and they pushed the progress much further, but still, it's not fully resolved. There are still open cases. I was just talking to my colleague at Technion and trying to find ways to pin down what essentially was left out in all the previous work.”
The missing ingredient was something in the field of spectral graph theory.
Graph theory is about learning properties of networks. Imagine a social network where people can be friends, so they are connected. The graph is just an abstract way to represent a network where people will become vertices or nodes. If two people are connected, they form an edge between those nodes, and this is what is called a graph.
Spectral graph theory is an interesting way to look at graphs, namely when one can represent a graph or translate a graph into a matrix and look at its eigenvalues. Spectral graph theory has turned out to be extremely useful in computer science, with applications such as Google’s superior PageRank algorithm.
Equiangular lines are examples of spherical codes, which are used to translate a message written in one form into a point on a high-dimensional sphere. This has important implications for information theory and communications.
“When we look at the equiangular lines problem, for each line, you can associate it with a vector. The vector is pointing in the direction of the line. If you start with a set of arrangements of lines separated by the same angles, and for each line you pick such a vector, these vectors will enjoy fantastic properties. You could imagine that these lines are originally arranged very symmetrically, and now the vectors you get out of those lines will also be quite symmetric – so that's one part of the story.
“From the information theoretical perspective, when you try to transmit information, you are basically sending vectors from one party to another. Let’s say I want to send an email to you, but at the end of the day, that’s just a sequence of zeros or ones. You can also just treat it as a vector, where the entries are just zeros and ones. And because of the transmission of information, noise could occur and the message could be corrupted. Information theorists try to come up with a selection of those vectors that are resilient to noise – and that translates to special properties of the selected vectors. One of the properties they would like to have is all the vectors be pairwise separated by the same angle. That’s the connection between the problem with the equiangular lines and coding theory,” said Jiang.
The team’s work provides a new result in spectral graph theory — that a bounded degree graph must have sublinear second eigenvalue multiplicity. The proof requires clever insights relating the spectrum of a graph with the spectrum of small pieces of graphs.
How did this great result come about — did the team of five researchers hover in front of a whiteboard for weeks on end, scribbling ideas and crossing them out? Not exactly. This was a summer research project (before COVID-19) where the team mostly interacted remotely using Slack, an instant-messaging app where users can share messages, images, internet links, videos and more.
Initially, the team had one in-person meeting where they got together and summarized the existing ideas, shared their perspectives of the problem and showed pitfalls they had encountered before.
“I would tell the group, when you read previous literature, you don't see what didn't work, right?” said Jiang. “When we read a math paper, it's always about what works — nobody's going to write an article about ‘these are the things I tried and I failed, sorry guys.’”
After that initial face-to-face meeting, the research team moved their entire discussion online over Slack.
“We still comment nowadays through Slack, and I feel like it's a fantastic way to communicate. Not only can we have a threaded discussion, and also we can just keep everything in one place. It's not like emails that you have to sort through. It keeps an ongoing record, which was great,” said Jiang.
After the problem percolated in the back of Jiang’s mind for a year, and then four other fresh minds were added, new ideas and directions started to flow.
“When you stop working on a problem for a while and then come back to it, definitely you get new ideas, and my explanation is that there are little people working (as he again gestures with his moving fingers behind his head), but I can't verify that,” said Jiang, smiling. “Definitely, when I look at the same problem once, twice, three times, I always get fresh ideas. But also, the other members in the group bring new ideas as well, so it's a combination of these two things.”
Over the summer, the team truly had fun working on the problem. We asked Jiang to discern what his specific contributions were to the solution.
“Let me actually be a bit conservative here, because I think the tradition in mathematics is that we try to push the frontier of human knowledge in a collective way. That's part of the reason why, for example, we don't sort authors by their contribution. I guess that's also why you see in other articles they try not to split the credits between the authors, and I want to keep that tradition, but also to answer your question," he said.
“I did have fun, and I can tell you at the beginning, we were a little bit stuck. We made some partial progress, but I guess by hitting those roadblocks we just learned a lot about what we needed at the end. And that was great experience, because at least for me personally, I feel like doing research is also about the experience. If you can get some results quite easily and straightforwardly, then you view the end result as less rewarding — but this is not like that. It’s quite challenging. When we did have a breakthrough and solved it, it was very rewarding and everyone was super excited.”
At 33 years old, Jiang is an old soul, respectful of the history and traditions of mathematics.
When he was a graduate student at Carnegie Mellon, he was inspired by the teaching of Professor Po-Shen Loh. Jiang was kind of lost in what he wanted, in which direction he wanted to do research. Loh was teaching something called extremal combinatorics, which is a subfield of combinatorics.
"His passion and enthusiasm just lit me up," said Jiang. "While listening to his teaching, you could feel that he just loves the subject, and it was entirely an inspirational experience."
Jiang believes in paying it forward in his own classroom. He strives to inspire his students because his own experience was like that.
“I feel if you can share your enthusiasm with the younger generation, that will just do a lot of good to the community," said Jiang.
“At the end of day, mathematics is about passing down the knowledge to the future generation, because once math is written in papers but nobody is reading them, it's dead. Working as a group, especially with undergrads or graduate students, has its own merits. In particular, the younger generation is also very creative, and they can just forget about the boundaries and limitations that our minds were trained with. They can break barriers."
