Professor receives NIH grant to investigate relationship between protein structure, health issues

Eleni Panagiotou
Proteins are very large molecules containing many amino acid residues linked together in specific sequence. They live in our bodies, in our cells, and encode function through their chemical composition and sequence. It is now well accepted that structured, governed dynamics modulate function, yet scientists still don’t know how a few changes in sequence, such as mutations, modify dynamics to alter function.
“If we knew that, we would be able to treat diseases and create new vaccines and maybe help prevent Alzheimer's disease and so on," said Eleni Panagiotou, assistant professor in Arizona State University's School of Mathematical and Statistical Sciences. "This is at the core of many, many problems in biology.”
Panagiotou has been awarded an interdisciplinary grant from the National Institutes of Health to investigate this problem. Together with co-principal investigator Banu Ozkan, professor and director of the Center for Biological Physics at ASU, she will study the relation between chemical composition, protein structure and protein function.
Despite the work of many researchers, the connection between sequence, structure and dynamics remains elusive. This is partly because there is no powerful method that can accurately quantify each amino-acid position’s contribution to structure and dynamics. Panagiotou's research team proposes to fill this gap by using an innovative, interdisciplinary method based on mathematical topology and physics-based protein dynamics modeling.
The idea comes from the fact that scientists know that chemical composition dictates protein structure, and then structure dictates protein function. Proteins are macromolecules, and the way they sit in three-dimensional space is related to their function. So as not to be random, they may fold into particular three-dimensional structures, by which the protein becomes biologically functional. Not all proteins fold, but many do, and other proteins misfold into disease-causing structures. The fact that structure is important for function and that structure is dictated by chemical composition enables researchers to use topology, which characterizes structure as an intermediate for making the connection between chemical composition and function.
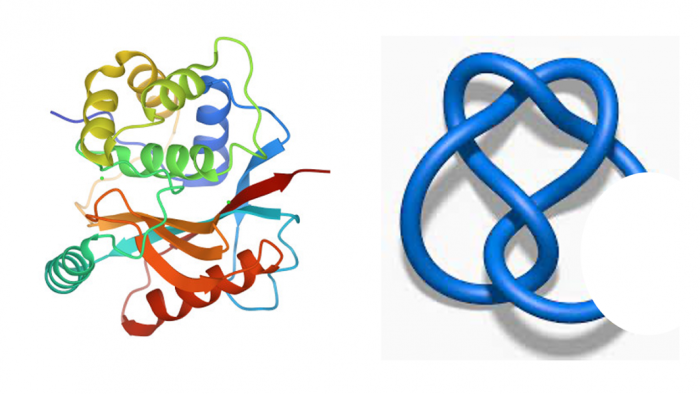
“Imagine the macromolecules could be like random filaments or like the cord from your headphones. As you take the headphones out of your bag, the cord appears to be randomly tangled,” Panagiotou explained. “But proteins are not really random. A protein may look like a random filament, but actually it has specific geometry and topology that we cannot assess by visual inspection alone, unless we employ some sophisticated mathematical tools.”
Panagiotou's guiding hypothesis is that the topological landscape of proteins governs conformational dynamics and that it can be modified with site-specific mutations.
Understanding the protein folding process has been an important challenge for computational biology since the late 1960s. People have thought of applying topology before to study proteins, but the tools they had been using could not address some of the aspects of proteins that are essential for characterizing them rigorously.
This innovative research project will create new mathematical tools for proteins using knot theory and combine them in a new molecular dynamics approach to proteins.
Knots in mathematics are simple, closed curves in space. That means things like shoelaces are not knots because they have non-connected endpoints. If you close them by tying them and gluing the ends together, then you have a mathematical knot. With knots, mathematicians can define all sorts of sophisticated things to tell them apart and learn things about them.
“Proteins are more like the shoelaces. They have open endpoints, and they may be very, very messy, making it hard to tell what knot you have when you can't even see the endpoints,” Panagiotou said. Her research group has developed mathematical tools that enable the characterization of all simple curves in three-dimensional space — which means those are open knots. They have extended the theory of knots to account for open knots in a rigorous way, using functions that behave well.
“I have been around biologists for 20 years now. Mathematicians and biologists have been trying to see if knot theory could be helpful in studying proteins. And they have been successful in finding that only 1% of known proteins contain knots,” Panagiotou said. “The new tools our team has developed now allow us to classify 94% of proteins.”
Experimentalists cannot see how proteins fold, how the process of folding is happening. They only see the end result of a protein. Mathematically, if researchers were trying to model this with all the details of chemical composition, with every amino acid in each specific position, it would be such a complicated problem that they likely could not solve it. Both mathematically and experimentally, it is a challenging problem to solve.
“We are using an innovative approach. Instead of trying to predict the structure through equations of motion, let's say, which would be an impossible mathematical problem, we are applying topological methods. We are using the topology of the folded protein, and we are learning from all folded proteins that exist in the protein data bank to understand the role of topologies in folding and protein function,” Panagiotou said.
Ozkan's physics group has done molecular dynamic simulations of proteins before, but has never combined this data with topological analysis. Preliminary data gives the promise that this would work.
An interdisciplinary relationship
When Panagiotou first arrived at ASU last fall, she spoke at a biological physics seminar organized by Ozkan. That meeting ignited the conversation during which the two identified the interdisciplinary project.
As part of ASU’s charter and goals, the university plans to expand its role as the leading global center for interdisciplinary research, discovery and development.
“All of ASU encourages interdisciplinary research, but I want to emphasize that the department of mathematics itself also encourages interdisciplinary research,” Panagiotou said. “I say that because many mathematics departments, in practice, don't so much. But ASU encourages this real, interdisciplinary research, and that is definitely very attractive to me.
“I think this is a more broadly accepted idea, that interdisciplinary research is needed. It is one of the National Science Foundation's 10 Big Ideas (Growing Convergence Research). I think interdisciplinary research in mathematics is appearing more and more.
“It is exciting that an area of what is called pure mathematics is finding such a direct application. This is 'unexpected applied mathematics,' because knot theory is a branch of topology, which traditionally is very theoretical."
The School of Mathematical and Statistical Sciences has a long history of success within their mathematical biology group, which includes both applied and pure mathematicians. Their areas of expertise include differential equations, dynamical systems, knot theory and probability and their applications to modeling in fields such as neuroscience, epidemiology, population biology and ecology, systems biology, soft matter (lipids, proteins) at interfaces, cancer, protein structure, protein folding, protein aggregation and polymer entanglement.
With the coming launch of ASU's School of Medicine and Advanced Medical Engineering, which will integrate clinical medicine, biomedical science and engineering, it will be even more critical for interdisciplinary research to become the norm.
“Both applied and pure mathematicians will be important collaborators for this innovative approach that will bring together health sciences from across the university to address complex health care problems,” said Donatella Danielli, foundation professor and director of the School of Mathematical and Statistical Sciences. “From more traditional pure mathematics to modern data science, all areas of mathematics are critical to the future success of scientific research.”
Panagiotou’s interdisciplinary project could lead to a breakthrough that would enable researchers to predict and modulate protein function based on structural dynamics, which will improve health outcomes and impact society.
The first step could lead to proposed experiments that will engineer proteins with desired function — engineer protein structure and thereby engineer protein function. If that is successful, the next step would be to engineer methods that will prevent proteins from malfunctioning.
“There are folded proteins in the cell, in the membrane of the virus, that are involved in the first stages of infection by controlling their shape. If we can attach something to keep them from making a specific movement or fold, we can engineer methods that can prevent infection," Panagiotou said. "That would lead to therapeutics for disease. For example, you may be able to engineer new vaccines for the SARS-CoV-2, the spike protein, and many other viruses.
“Thousands of scientists have been working on protein folding, but no one has done it the way we are doing it. A few have combined topology before, but no one has done it with the novel tools that we have developed, which are significant improvements of what existed before.”
In addition, Panagiotou has received a pilot grant from the Arizona Alzheimer’s Consortium to create some preliminary data that shows whether the topology of tau proteins can predict their misfolding. The diseases that her team are looking at for this project are called tauopathies — neurodegenerative disorders characterized by the deposition of abnormal tau protein in the brain.
“We want to use tools from topology to first characterize the different shapes. We can look at them and say they look different, but how can we quantify it? That is a first step in making the problem mathematical, to characterize and classify different tauopathies,” Panagiotou said.
“The second part is to predict where along these proteins which amino acid may be essential for misfolding. If we get preliminary results that are backed up by existing experiments, our goal is to propose new experiments to test our hypothesis about particular locations we predict are important for this misfolded structure. In the long term, if those experiments work, then we would try to create therapeutics that can act at specific sites to prevent tau proteins from misfolding.”
More Science and technology

Cracking the code of online computer science clubs
Experts believe that involvement in college clubs and organizations increases student retention and helps learners build valuable…
Consortium for Science, Policy & Outcomes celebrates 25 years
For Arizona State University's Consortium for Science, Policy & Outcomes (CSPO), recognizing the past is just as important as…

Hacking satellites to fix our oceans and shoot for the stars
By Preesha KumarFrom memory foam mattresses to the camera and GPS navigation on our phones, technology that was developed for…