Upon first thought, the idea of math combating violent crime seems unlikely, at best. Can an algorithm be more effective than a SWAT team with a battering ram?
Yes. By mathematically modeling systems, pressure points can be identified and squeezed until the system changes or collapses. Instead of bludgeoning a problem into line, it’s like finding a carotid artery and pinching it until the opponent collapses.
That’s the idea behind the establishment of a mathematical modeling center at an El Salvador university named after Arizona State University professor Carlos Castillo-Chavez late last month.
This center will focus on researching the main problems of the country, particularly, in the short term, designing models for addressing violence and its prevention. The objective is to analyze data related to the issues of violence, insecurity and their derivatives.
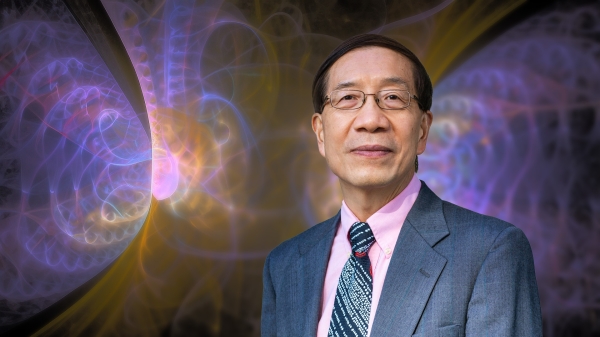
Francisco Gavidia University has created the Center for Mathematical Modeling “Carlos Castillo-Chavez” in honor of the Mexican-American scientist at ASU. Dr. Castillo-ChavezDr. Castillo-Chavez is a University Regents’ Professor and Joaquin Bustoz Jr. Professor of mathematical biology in the School of Human Evolution and Social Change in the College of Liberal Arts and Sciences, distinguished sustainability scientist in the Julie Ann Wrigley Global Institute of Sustainability, and executive director of the Simon A. Levin Mathematical, Computational and Modeling Sciences Center. will support the center’s technical implementation and assist in the development of collaborative research with ASU and other institutions throughout Latin America.
Mathematics can’t solve the problem of violence per se, but mathematical models are key components of interdisciplinary efforts aimed at quantifying the impact of violence, Castillo-Chavez said.
Models can “help identify what are the key mechanisms that facilitate the spread of violence in communities and regions to the point that it may alter societal norms,” he said. “They can also help you identify the pressure points of the system; that is, what are the key nodes that, if modified, would have the most impact on the reduction of crime.”
“A key problem in El Salvador involves gangs. Can we model their dynamics? Recruitment? Progression? ... What would happen if we reduce recruitment — somehow make it harder? By 10 percent? By 20 percent? By 30 percent? At what point does it make a difference?”
— ASU mathematician Carlos Castillo-Chavez
The process is to ask questions, build scenarios in the form of experiments, define constraints and proceed to address the problem.
“A key problem in El Salvador involves gangs,” Castillo-Chavez said. “Can we model their dynamics? Recruitment? Progression? Until they become hard-core? What are the key pressure points? Recruitment? What would happen if we reduce recruitment — somehow make it harder? By 10 percent? By 20 percent? By 30 percent? At what point does it make a difference? What if recidivism is high? How do we reduce the levels of recidivism? Can we do it if individuals within the environment that they live in are dominated by gangs?”
What Castillo-Chavez is looking for is the “tipping point,” an idea explained by journalist Malcolm Gladwell in his 2000 book of the same name.
It’s “the idea that social problems behave like infectious agents,” Gladwell said in his original article on the topic in the New Yorker magazine in 1996. “This is the fundamental lesson of nonlinearity. When it comes to fighting epidemics, small changes — like bringing new infections down to thirty thousand from forty thousand — can have huge effects. And large changes — like reducing new infections to fifty thousand from a hundred thousand — can have small effects. It all depends on when and how the changes are made.”
Castillo-Chavez also wrote about applying epidemiology modeling to life and social sciences in a 2011 paper.
“The concept of threshold or tipping point, a mathematical expression that characterizes the conditions needed for the occurrence of a drastic transition between epidemiological states, is central to the study of the transmission dynamics and control of diseases,” he wrote. “Epidemiological thinking has transcended the realm of epidemiological modeling and in the process, it has found applications to the study of dynamic social process where contacts between individuals facilitate the build-up of communities that can suddenly (tipping point) take on a life of their own.”
The main idea is to use mathematics to model the processes that produce violence, and then use the model to figure out how to alter these processes by whatever interventions are appropriate to reduce violence, said Ed Kaplan, the main speaker at the center’s opening.
Kaplan is the William N. and Marie A. Beach Professor of Management Science at the Yale School of Management, professor of public health at the Yale School of Medicine, and professor of engineering in the Yale School of Engineering and Applied Science.
“Reducing gang violence largely reduces to reducing the population of gang members; fewer gang members, fewer people to commit violent crimes,” he said. “There are two basic ways to reduce the size of any population: increase the rate that people leave, or reduce the rate that people join.
“Increasing the rate that people leave is essentially the job of law enforcement — and the many arrests and incarcerations of Salvadoran gang members testify to this approach. But even more important is starving gangs of new members. Here the key ingredients are education and job opportunities.
“A mathematical model can help relate the impact of investments in such diversion programs as well as the impact of different levels of enforcement on the resulting gang population, and show what the most cost-effective interventions are.”
Top photo by Aleksandar Milosevic/Freeimages.com
More Science and technology
Extreme HGTV: Students to learn how to design habitats for living, working in space
Architecture students at Arizona State University already learn how to design spaces for many kinds of environments, and now they can tackle one of the biggest habitat challenges — space architecture…
Human brains teach AI new skills
Artificial intelligence, or AI, is rapidly advancing, but it hasn’t yet outpaced human intelligence. Our brains’ capacity for adaptability and imagination has allowed us to overcome challenges and…

Doctoral students cruise into roles as computer engineering innovators
Raha Moraffah is grateful for her experiences as a doctoral student in the School of Computing and Augmented Intelligence, part of the Ira A. Fulton Schools of Engineering at Arizona State University…
